int 1x {6 (logx) ^2+ 7logx + 2 } d x =
Por um escritor misterioso
Last updated 10 janeiro 2025

Click here:point_up_2:to get an answer to your question :writing_hand:6 the value of int frac e 5 log x
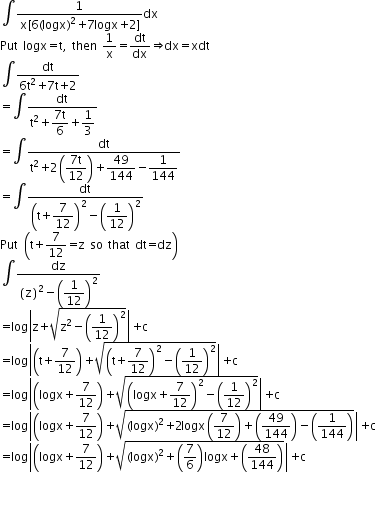
I=?1/[x{6(log x)^2 + 7(log x) + 2}] dx - vrcb5wkk
Equação Logarítmica - Brasil Escola

JEE Advanced 2021 Paper 2 Maths Question Paper - Download PDF

integrate dx/x(6(logx) ^2 +7logx +2) - rxhomeyy

Solved Evaluate the integrals 65. L log2 (x + 2) x + 2 dx

int 1/(x( 6 ( log x)^2 + 7 log x + 2) dx`

Telugu] Match the following. I. int (1)/(9+x^(2))dx= a) (1)

Example 38 - Evaluate [log (log x) + 1 / (log x)2 ] dx - Examples

int 1/(x( 6 ( log x)^2 + 7 log x + 2) dx`

int 1/(x( 6 ( log x)^2 + 7 log x + 2) dx
Evaluate the following integral : ∫1/(x{6(logx)^2+7logx+2})dx - Sarthaks eConnect

Ex 7.6, 15 - Integrate (x2 + 1) log x - Chapter 7 NCERT - Ex 7.6
Solving Logarithmic Equations – Explanation & Examples

Highlighted XII_M-2.pdf - Maths - Notes - Teachmint

PDF) Tail estimates for the Brownian excursion area and other Brownian areas
Recomendado para você
-
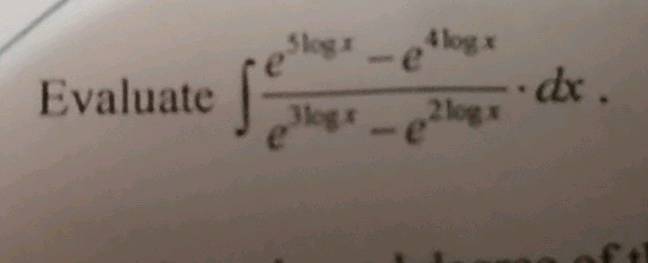 Evaluate: int e^log√(x)xdx10 janeiro 2025
Evaluate: int e^log√(x)xdx10 janeiro 2025 -
 e ^ { 5 log x } - e ^ { 4 log x } ) ( e ^ { 3 log x } - e ^ { 2 log x } d x )10 janeiro 2025
e ^ { 5 log x } - e ^ { 4 log x } ) ( e ^ { 3 log x } - e ^ { 2 log x } d x )10 janeiro 2025 -
Solved s. Find the first derivative of X - 1/X2 (FROM FIRST10 janeiro 2025
-
 Campeonato de futebol Amador de Itatiba chega às quartas de final e briga pela artilharia fica empolgante - Itatiba Hoje10 janeiro 2025
Campeonato de futebol Amador de Itatiba chega às quartas de final e briga pela artilharia fica empolgante - Itatiba Hoje10 janeiro 2025 -
 Itatiba EC/PMI joga neste sábado pelas semifinais da Copa Itatiba de Handebol Masculino - Jornal de Itatiba10 janeiro 2025
Itatiba EC/PMI joga neste sábado pelas semifinais da Copa Itatiba de Handebol Masculino - Jornal de Itatiba10 janeiro 2025 -
S.C. Palermo - Itatiba Sp10 janeiro 2025
-
%2008_31_06_e51ca4f3.jpg) Notícia - Sub 15 feminino - Prefeitura Municipal de Albertina10 janeiro 2025
Notícia - Sub 15 feminino - Prefeitura Municipal de Albertina10 janeiro 2025 -
What is the integration of this function [math]I=\displaystyle \int \dfrac{e^{6 \log x}-e^{5 \log x}}{e^{4 \log x}-e^{3 \log x}} \,d x[/math]? - ISI/CMI World - Quora10 janeiro 2025
-
 Strength gain trend over time for clean sand and FCR.10 janeiro 2025
Strength gain trend over time for clean sand and FCR.10 janeiro 2025 -
 ᴛᴀɴʏᴀ🍁:) on Instagram: 😂 . #BornOnInstagram #exammemes #examstress #studymemes #studymotivation #schoolmemories #medicalstudents #commercestudents #engineeringstudents #examsfinished #originalcontent #desiproblems#overthinking #relatable10 janeiro 2025
ᴛᴀɴʏᴀ🍁:) on Instagram: 😂 . #BornOnInstagram #exammemes #examstress #studymemes #studymotivation #schoolmemories #medicalstudents #commercestudents #engineeringstudents #examsfinished #originalcontent #desiproblems#overthinking #relatable10 janeiro 2025
você pode gostar
-
 Patrulha Canina Almanaque De Atividades Para Colorir: Patrulha Canina Almanaque De Atividades Para Colorir, De Onl. Editora Online, Capa Mole, Edição 1 Em Português, 202210 janeiro 2025
Patrulha Canina Almanaque De Atividades Para Colorir: Patrulha Canina Almanaque De Atividades Para Colorir, De Onl. Editora Online, Capa Mole, Edição 1 Em Português, 202210 janeiro 2025 -
 Carrinho de Controle Remoto Escala 120 até 25 km por hora, DEERC, Vermelho - Blumenau10 janeiro 2025
Carrinho de Controle Remoto Escala 120 até 25 km por hora, DEERC, Vermelho - Blumenau10 janeiro 2025 -
 Fish Feel Pain. Now What?10 janeiro 2025
Fish Feel Pain. Now What?10 janeiro 2025 -
 One Room, Hiatari Futsuu, Tenshi-tsuki. - Statistics10 janeiro 2025
One Room, Hiatari Futsuu, Tenshi-tsuki. - Statistics10 janeiro 2025 -
 what is Retinoblastoma ? - Amrita Medical Center10 janeiro 2025
what is Retinoblastoma ? - Amrita Medical Center10 janeiro 2025 -
 10 Resident Evil Games That Deserve Remakes10 janeiro 2025
10 Resident Evil Games That Deserve Remakes10 janeiro 2025 -
 Edição Bedrock beta 1.20.0.20 - Minecraft Wiki10 janeiro 2025
Edição Bedrock beta 1.20.0.20 - Minecraft Wiki10 janeiro 2025 -
 Kano Evolution by TheShakifanN16 on DeviantArt10 janeiro 2025
Kano Evolution by TheShakifanN16 on DeviantArt10 janeiro 2025 -
 Creeper Overhaul Mod / Minecraft 1.1810 janeiro 2025
Creeper Overhaul Mod / Minecraft 1.1810 janeiro 2025 -
 IPN on X: / X10 janeiro 2025
IPN on X: / X10 janeiro 2025

