1) Sketch the parabola, and lable the focus, vertex and directrix. a) (y - 1)^2 = -12(x + 4) b) i) y^2 - 6y -2x + 1 = 0, ii) y =
Por um escritor misterioso
Last updated 03 novembro 2024


HELP! ONLY IF YOU KNOW THE ANSWER also this goes with the other question I asked Write the standard
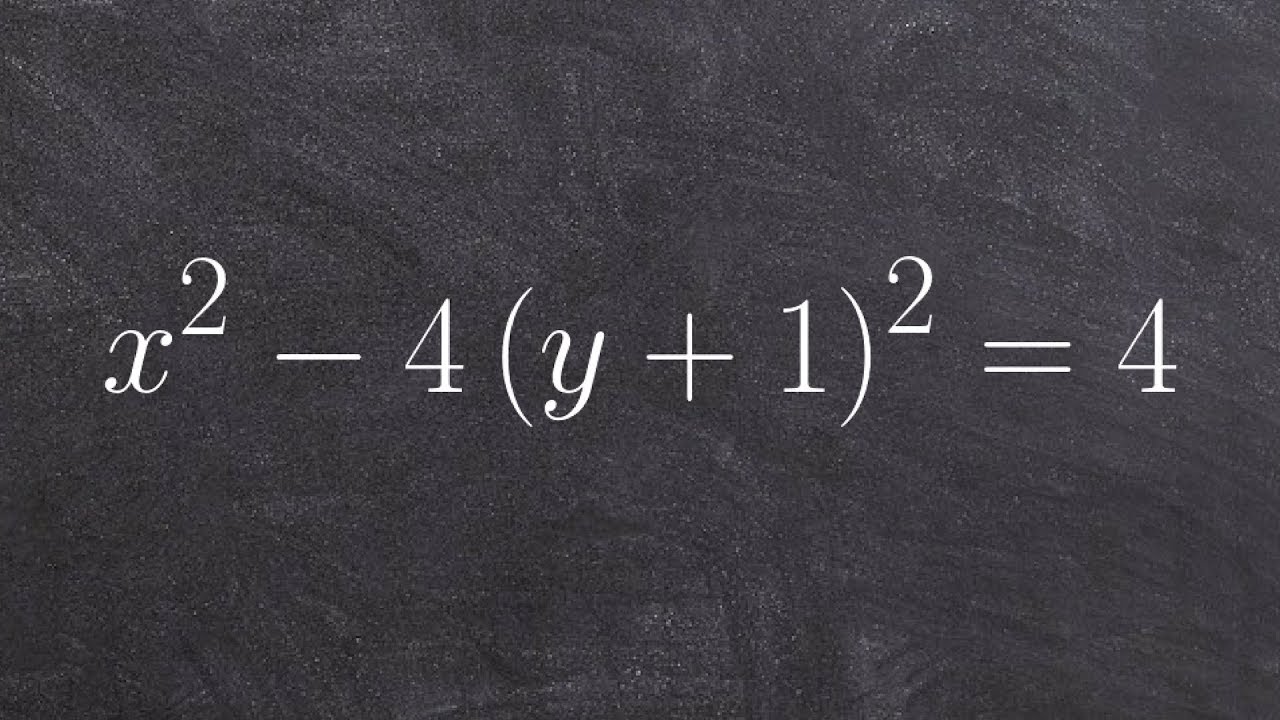
how to sketch the graph of a hyperbola in conic sections
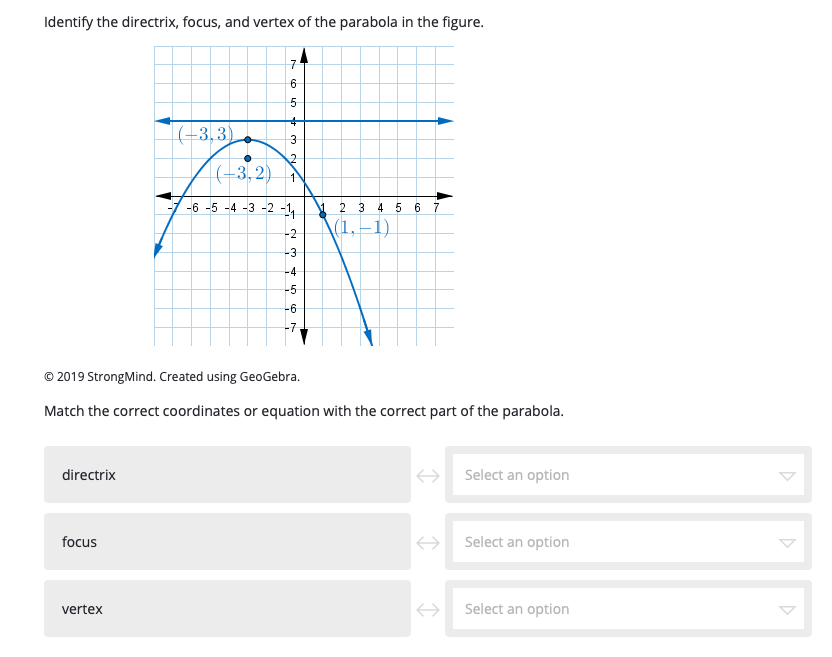
Answered: Identify the directrix, focus, and…
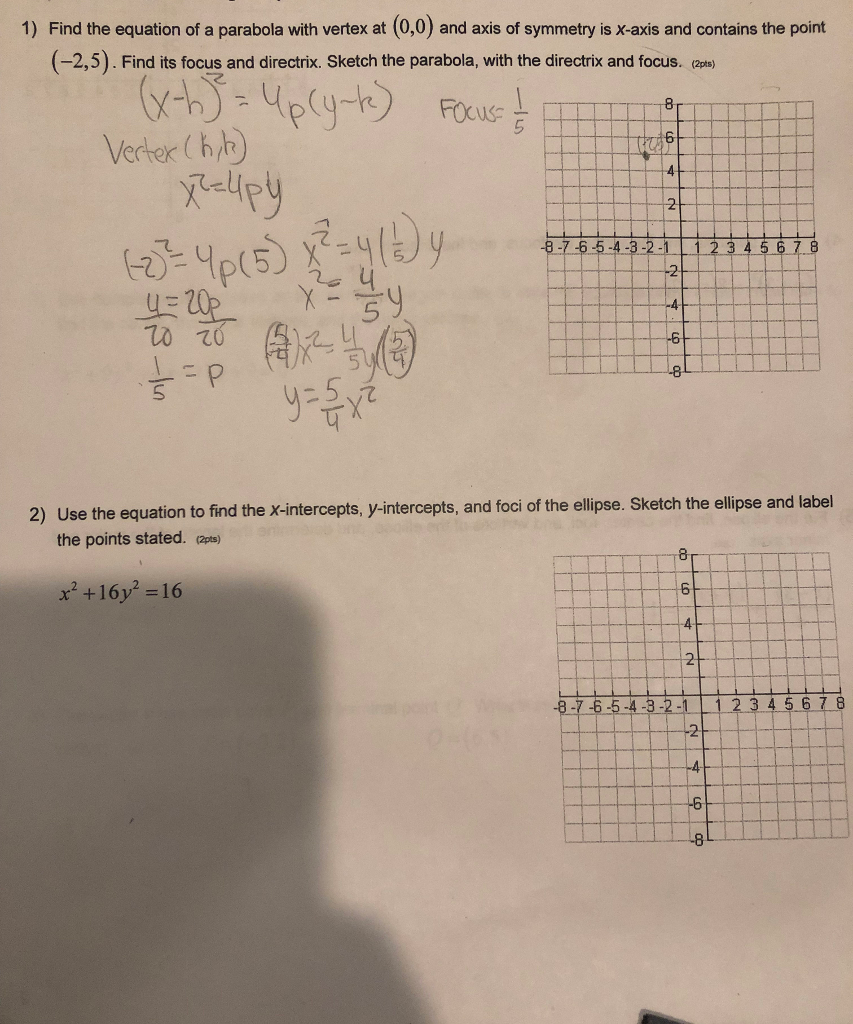
Solved Find the equation of a parabola with vertex at (0,0)
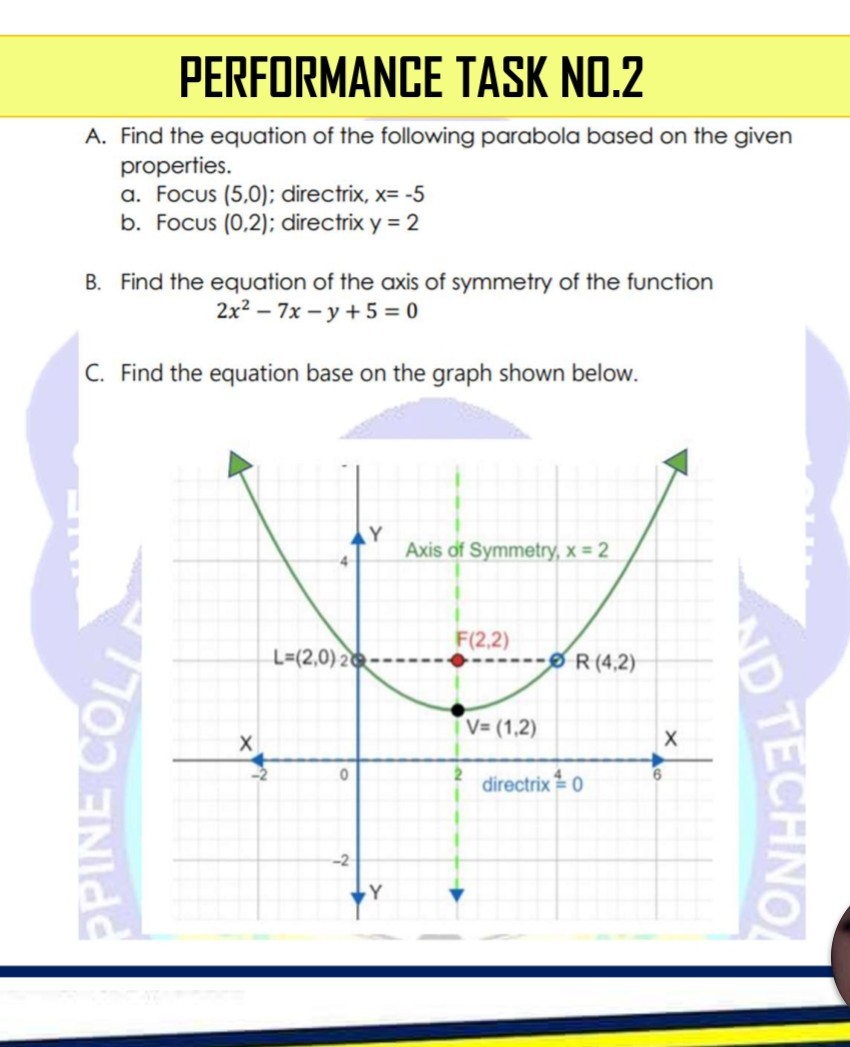
SOLVED: PERFIRMANCE TASK NU.2 A. Find the equation of the following parabola based on the given properties. a. Focus (5,0); directrix, x=-5 b. Focus (0,2); directrix y=2 B. Find the equation of

Find the vertex, focus, and directrix of each parabola. Grap
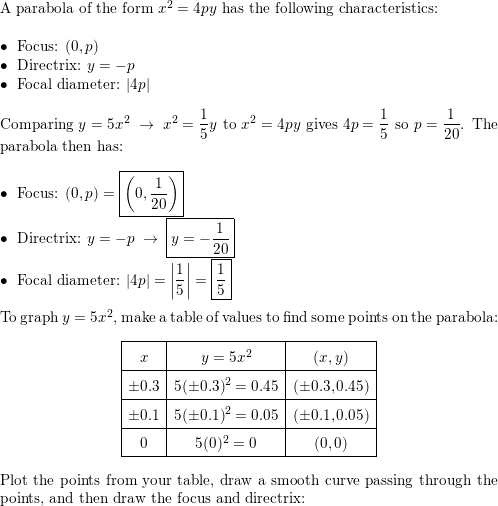
Find the focus, directrix, and focal diameter of the parabol
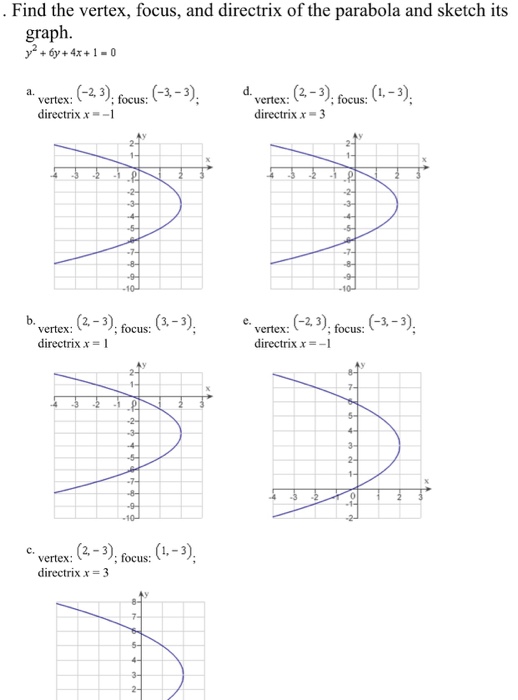
Solved Find the vertex, focus, and directrix of the parabola
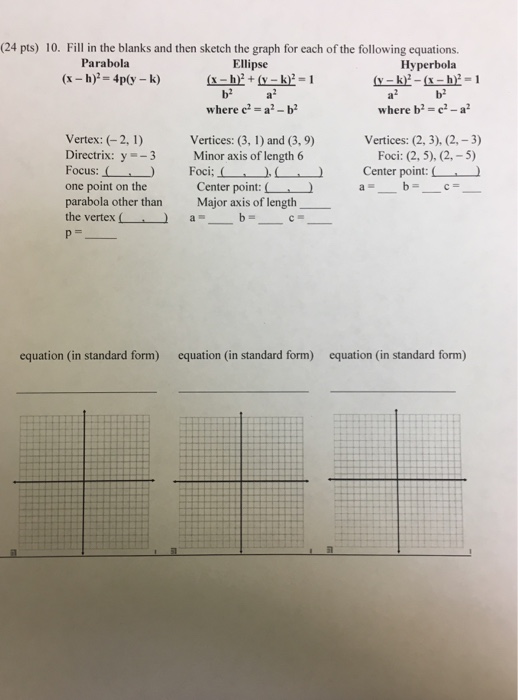
Solved Fill in the blanks and then sketch the graph for each
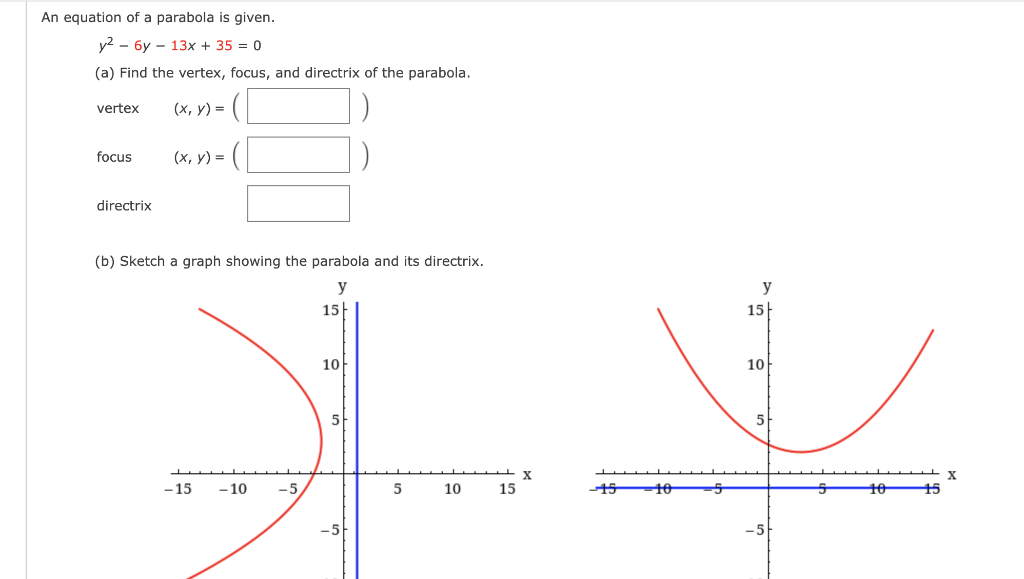
Solved An equation of a parabola is given. y2−6y−13x+35=0

Conic sections: Analyzing Conic Sections with the Algebraic Method - FasterCapital
Recomendado para você
-
 Learning DirectX 12 – Lesson 1 – Initialize DirectX 1203 novembro 2024
Learning DirectX 12 – Lesson 1 – Initialize DirectX 1203 novembro 2024 -
 DirectX 12 Technology03 novembro 2024
DirectX 12 Technology03 novembro 2024 -
 AMD DirectX® 12 (DX12) Technology03 novembro 2024
AMD DirectX® 12 (DX12) Technology03 novembro 2024 -
 DirectX 12 Will Allow Multi-GPU Between GeForce And Radeon : r/Games03 novembro 2024
DirectX 12 Will Allow Multi-GPU Between GeForce And Radeon : r/Games03 novembro 2024 -
 Directx 2007 Free - Colaboratory03 novembro 2024
Directx 2007 Free - Colaboratory03 novembro 2024 -
 All NEW Fortnite Chapter 2 Settings! - DirectX 12, Console Motion Blur, & More!03 novembro 2024
All NEW Fortnite Chapter 2 Settings! - DirectX 12, Console Motion Blur, & More!03 novembro 2024 -
 AMD 780G: Best Ever Integrated Mainstream Chipset? - Silent PC Review03 novembro 2024
AMD 780G: Best Ever Integrated Mainstream Chipset? - Silent PC Review03 novembro 2024 -
![Square Enix Unveils Impressive WITCH CHAPTER 0 [cry] DirectX 12 Tech Demo At Microsoft BUILD](https://imageio.forbes.com/blogs-images/marcochiappetta/files/2015/04/witch-cry-2.jpg?height=400&width=711&fit=bounds) Square Enix Unveils Impressive WITCH CHAPTER 0 [cry] DirectX 12 Tech Demo At Microsoft BUILD03 novembro 2024
Square Enix Unveils Impressive WITCH CHAPTER 0 [cry] DirectX 12 Tech Demo At Microsoft BUILD03 novembro 2024 -
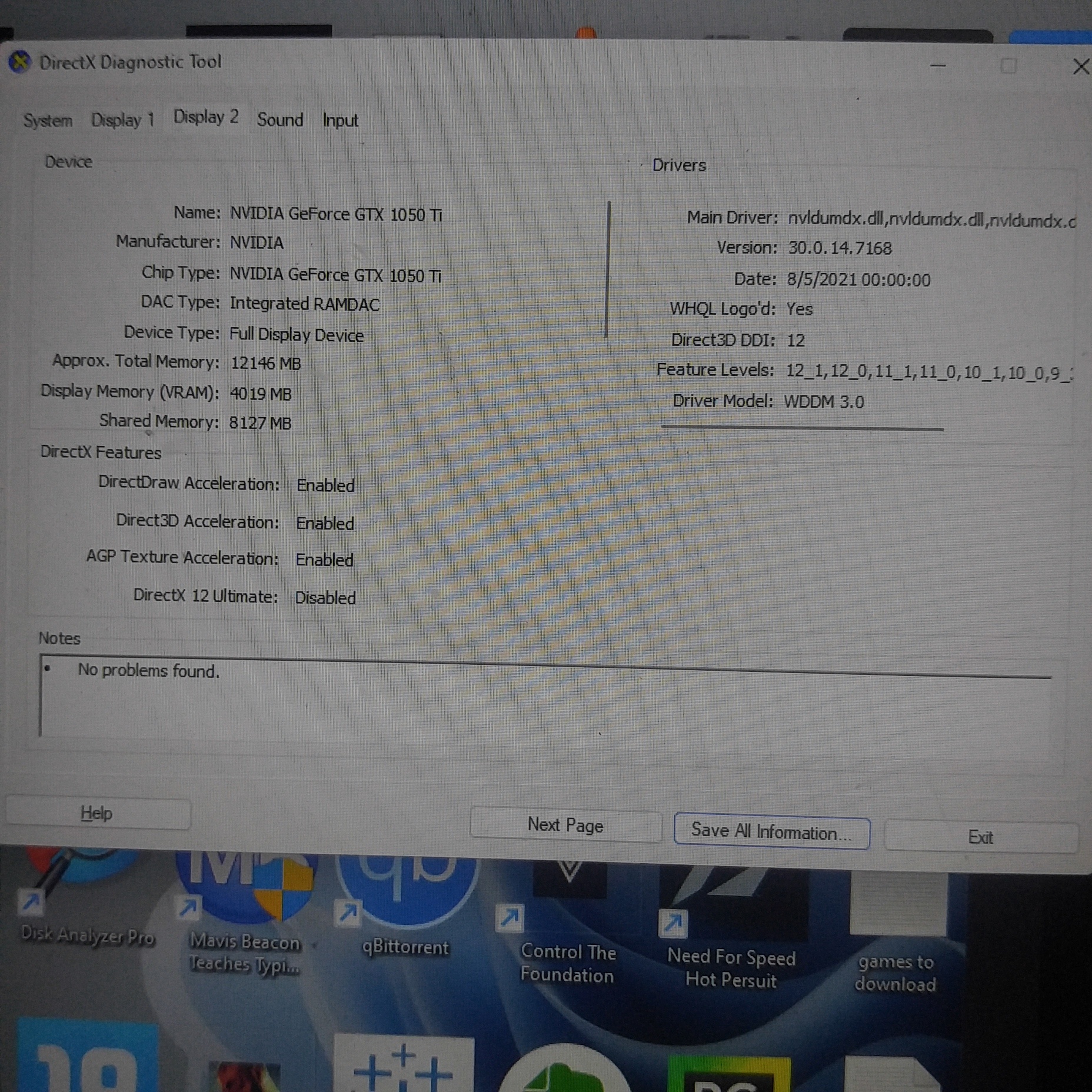
Dxdiag shows DirectX 12 Ultimate as Disabled. How to enable? - Microsoft Community03 novembro 2024
-
 DirectX 11 vs. DirectX 12 - Test in 10 Games on RTX 3060 Ti (Which is Better?)03 novembro 2024
DirectX 11 vs. DirectX 12 - Test in 10 Games on RTX 3060 Ti (Which is Better?)03 novembro 2024
você pode gostar
-
 Jordan x Teyana Taylor Women's Jumpsuit.03 novembro 2024
Jordan x Teyana Taylor Women's Jumpsuit.03 novembro 2024 -
 Modern Warfare 3 Early Access Plagued with Issues - FandomWire03 novembro 2024
Modern Warfare 3 Early Access Plagued with Issues - FandomWire03 novembro 2024 -
 Reaper 2: Auto Quest, Auto Skill & More Scripts03 novembro 2024
Reaper 2: Auto Quest, Auto Skill & More Scripts03 novembro 2024 -
 Crossy Road Indie Game Marketing: At Home Update – VGCD Academy03 novembro 2024
Crossy Road Indie Game Marketing: At Home Update – VGCD Academy03 novembro 2024 -
 How Many People Are In the World In 2023? - Parade03 novembro 2024
How Many People Are In the World In 2023? - Parade03 novembro 2024 -
 Jornadas Pokémon - O Vício03 novembro 2024
Jornadas Pokémon - O Vício03 novembro 2024 -
 unpopular (or outright bad) games that you enjoy - General Discussion - Giant Bomb03 novembro 2024
unpopular (or outright bad) games that you enjoy - General Discussion - Giant Bomb03 novembro 2024 -
 I Love You In Chinese // 37 Killer Phrases (To Express Your True03 novembro 2024
I Love You In Chinese // 37 Killer Phrases (To Express Your True03 novembro 2024 -
Gold Diggers03 novembro 2024
-
Mega Pokemons Evolutions - Fan made03 novembro 2024